• Main
• Contact
• Education
• Research
• Publications
• Talks
• Students
• Images
• Links

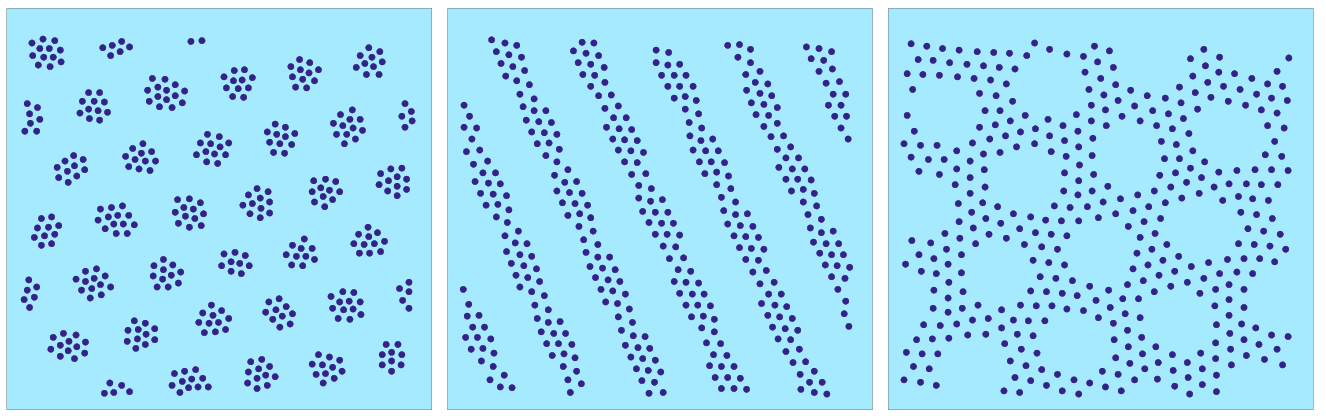
Competing long-range/short-range interactions

Two-dimensional systems in which there is a competition between long-range repulsion and short range attraction exhibit a remarkable variety of patterns such as stripes, bubbles, and labyrinths. Such systems include magnetic films, Langmuir monolayers, polymers, gels, and water-oil mixtures. It has been proposed that similar competing interactions can arise in two-dimensional electron systems leading to stripes, clumps, and liquid crystalline electron states. Stripe and other charge-ordered phases in metal oxides are sometimes modeled as systems with competing long range repulsion and short range attraction. In many of these systems quenched disorder from the underlying substrate may be present; however, it is not known how this disorder would affect the structure and dynamics of these systems. Quenched disorder can strongly alter the transport properties, producing a pinning effect in which a finite driving force must be applied before net motion occurs.

Preprints:

1. Ratchet effects in cyclic pattern formation systems with competing interactions
   C. Reichhardt and C.J.O. Reichhardt
   Ratchet effects can appear for particles interacting with an asymmetric potential under ac driving or for a thermal system in which a substrate is periodically flashed. Here, we show that a new type of collective ratchet effect can arise for a pattern-forming system coupled to an asymmetric substrate when the interaction potential between the particles is periodically oscillated in order to cycle the system through different patterns. We consider particles with competing short-range attraction and long-range repulsion subjected to time-dependent oscillations of the ratio between the attractive and repulsive interaction terms, which causes the system to cycle periodically between crystal and bubble states. In the presence of the substrate, this system exhibits both a positive and a reversed ratchet effect, and we show that there is a maximum in the ratchet efficiency as a function of interaction strength, ac drive frequency, and particle density. Our results could be realized for a variety of pattern-forming systems on asymmetric substrates where the pattern type or particle interactions can be oscillated. arXiv
   

Papers:

1. Driven probe particle dynamics in a bubble forming system
   C. Reichhardt and C.J.O. Reichhardt
   J. Chem. Phys., in press (2025). arXiv
   

   
   

2. Active microrheology and dynamic phases for pattern forming systems with competing interactions
   C. Reichhardt and C.J.O. Reichhardt
   Phys. Rev. E 111, 055406 (2025). arXiv
   

   
   

3. Directional locking and hysteresis in stripe and bubble forming systems on one-dimensional periodic substrates with a rotating drive
   C. Reichhardt and C.J.O. Reichhardt
   Phys. Rev. E 111, 054119 (2025). arXiv
   

   
   

4. Stripe and bubble ratchets on asymmetric substrates
   C. Reichhardt and C.J.O. Reichhardt
   Phys. Rev. Res. 6, 043290 (2024). arXiv
   

   
   

5. Peak effect and dynamics of stripe and pattern forming systems on a periodic one dimensional substrate
   C. Reichhardt and C.J.O. Reichhardt
   Phys. Rev. E 109, 054606 (2024). arXiv
   

   
   

6. Sliding dynamics for bubble phases on periodic modulated substrates
   C. Reichhardt and C.J.O. Reichhardt
   Phys. Rev. Res. 6, 023116 (2024). arXiv
   
   
7. Structural transitions and hysteresis in clump- and stripe-forming systems under dynamic compression
   D. McDermott, C.J. Olson Reichhardt, and C. Reichhardt
   Soft Matter 12, 9549 (2016). arXiv

   
   

8. Stripe systems with competing interactions on quasi-one-dimensional periodic substrates
   D. McDermott, C.J. Olson Reichhardt, and C. Reichhardt
   Soft Matter 10, 6332 (2014). arXiv
   

   
   

9. Ordering of colloids with competing interactions on quasi-one-dimensional periodic substrates
   C. Reichhardt, D. McDermott, and C.J. Olson Reichhardt
   Proc. SPIE 9164, Optical Trapping and Optical Micromanipulation XI, 916420 (2014).
   

   
   

10. Static and dynamic phases for magnetic vortex matter with attractive and repulsive interactions
    J.A. Drocco, C.J. Olson Reichhardt, C. Reichhardt, and A.R. Bishop
    J. Phys.: Condens. Matter 25, 345703 (2013).
    

    
    

11. Statics and dynamics of vortex matter with competing repulsive and attractive interactions
    C. Reichhardt, J. Drocco, C.J. Olson Reichhardt, and A.R. Bishop
    J. Supercond. Nov. Magn. 26, 2041 (2013). arXiv

    
    

12. The effect of pinning on vortex states with attractive and repulsive interactions
    C. Reichhardt, J. Drocco, C.J. Olson Reichhardt, and A.R. Bishop
    Physica C 479, 15 (2012).
    

    
    

13. Statics and dynamics of wetting-dewetting transitions for particles with attractive interactions on periodic substrates
    J.A. Drocco, C. Reichhardt, C.J. Olson Reichhardt, and A.R. Bishop
    Proc. SPIE 8458, Optical Trapping and Optical Micromanipulation IX, 84581J (2012).
    

    
    

14. Anisotropic sliding dynamics, peak effect, and metastability in stripe systems
    C.J. Olson Reichhardt, C. Reichhardt, and A.R. Bishop
    Phys. Rev. E 83, 041501 (2011). arXiv

    
    

15. Structural transitions, melting, and intermediate phases for stripe- and clump-forming systems
    C.J. Olson Reichhardt, C. Reichhardt, and A.R. Bishop
    Phys. Rev. E 82, 041502 (2010). arXiv

    
    

16. Commensurate and incommensurate checkerboard charge ordered states
    C. Reichhardt, C.J. Olson Reichhardt, and A.R. Bishop
    Physica C 460-462, 1178 (2007).
    

    
    

17. Noise and hysteresis in charged stripe, checkerboard, and clump forming systems
    C. Reichhardt, C.J. Olson Reichhardt, and A.R. Bishop
    Proc. SPIE 6600, Noise and Fluctuations in Circuits, Devices, and Materials, 66001B (2007).
    

    
    

18. Structure and fragmentation in colloidal artificial molecules and nuclei
    C.J. Olson Reichhardt, C. Reichhardt, and A.R. Bishop
    Eur. Phys. J. E 22,11 (2007). arXiv

    
    

19. Hysteresis and noise in stripe- and clump- forming systems
    C. Reichhardt, C.J. Olson Reichhardt, and A.R. Bishop
    Europhys. Lett. 72, 444 (2005). arXiv

    
    

20. Dynamics and melting of stripes, crystals, and bubbles with quenched disorder
    C.J. Olson Reichhardt, C. Reichhardt, I. Martin, and A.R. Bishop
    Physica D 193, 303 (2004). arXiv

    
    

21. Fibrillar templates and soft phases in systems with short-range dipolar and long-range interactions
    C.J. Olson Reichhardt, C. Reichhardt, and A.R. Bishop
    Phys. Rev. Lett. 92, 016801 (2004). arXiv

    
    

22. Effect of field-effect transistor geometry on charge ordering of transition-metal oxides
    C.J. Olson Reichhardt, C. Reichhardt, D.L. Smith, and A.R. Bishop
    Phys. Rev. B 68, 033101 (2003). arXiv

    
    

23. Dynamical ordering of driven stripe phases in quenched disorder
    C. Reichhardt, C.J. Olson Reichhardt, I. Martin, and A.R. Bishop
    Phys. Rev. Lett. 90, 026401 (2003). arXiv

    
    

24. Depinning and dynamics of systems with competing interactions in quenched disorder
    C. Reichhardt, C.J. Olson, I. Martin, and A.R. Bishop
    Europhys. Lett. 61, 221 (2003). arXiv