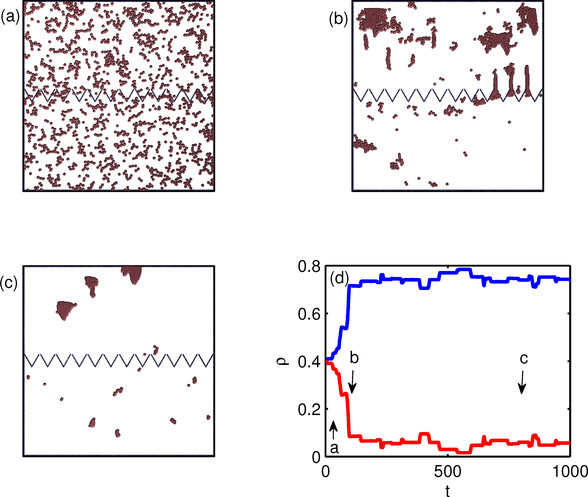
 Figure 2:
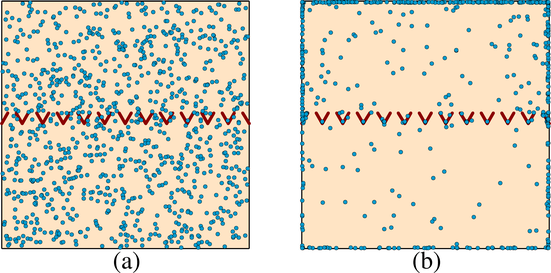
The particle positions (dots) for the same geometry
in Fig. 1 but for a flocking model.
Here the particle exclusion radius is small, re = 0.07.
(a) An early time configuration with equal numbers
of particles on both sides of the funnel barrier.
(b) At a later time, particles start to accumulate in the upper chamber.
Particles enter the upper chamber in the form of flocks that
squeeze through the funnel tips.
(c) In steady state, most of the particles
are in the upper chamber.
(d) The density ρ of particles in the upper (top blue curve)
and lower (bottom red curve) chambers
vs time. The letters indicate the
times illustrated in panels a, b, and c.
Figure 2:
The particle positions (dots) for the same geometry
in Fig. 1 but for a flocking model.
Here the particle exclusion radius is small, re = 0.07.
(a) An early time configuration with equal numbers
of particles on both sides of the funnel barrier.
(b) At a later time, particles start to accumulate in the upper chamber.
Particles enter the upper chamber in the form of flocks that
squeeze through the funnel tips.
(c) In steady state, most of the particles
are in the upper chamber.
(d) The density ρ of particles in the upper (top blue curve)
and lower (bottom red curve) chambers
vs time. The letters indicate the
times illustrated in panels a, b, and c.
|