 |
Center for Nonlinear Studies |
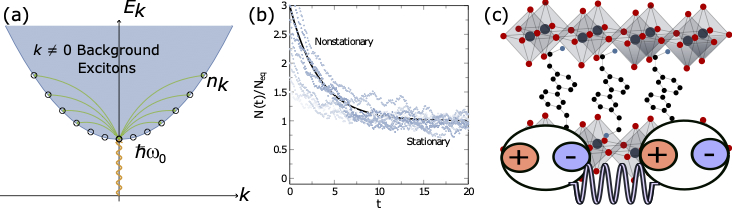
Modern multi-dimensional spectroscopy offers a unique look "under the hood" allowing us to probe the dynamics of excitons in semiconducting systems. In this talk, I shall review our recent quantum stochastic model for spectroscopic lineshapes in a co-evolving and non-stationary background population of excitations. Starting from a field theory description for interacting bosonic excitons, we derive a reduced model whereby optical excitons are coupled to an incoherent background via scattering as mediated by their screened Coulomb coupling. Such processes include intra- and inter-valley excitons. The Heisenberg equations of motion for the optical excitons are then driven by an auxiliary stochastic population variable, which we take as the solution of an Ornstein�Uhlenbeck process. Here we discuss an overview of the theoretical techniques we have developed to predict coherent non-linear spectroscopic signals. We show how direct (Coulomb) and exchange coupling to the bath give rise to distinct spectral signatures such as phase-scrambling, excitation-induced dephasing, and excitation-induced shifts. We also discuss mathematical limits on inverting spectral signatures to extract the background density of states.
Host: Abhijith Jayakumar (T-5)