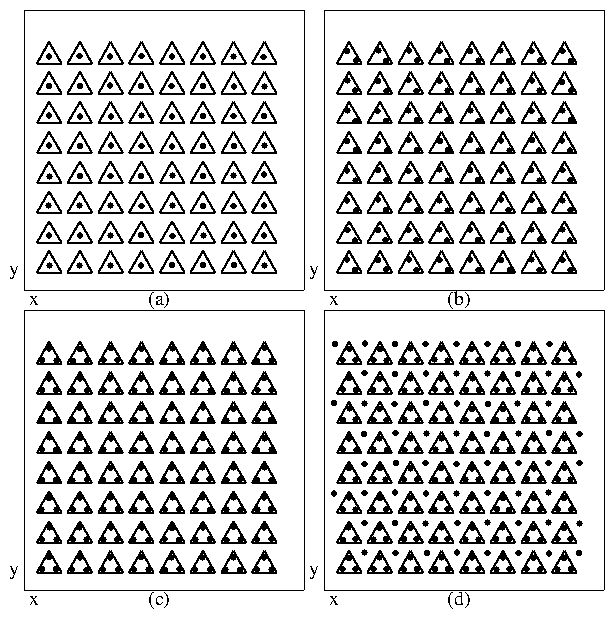
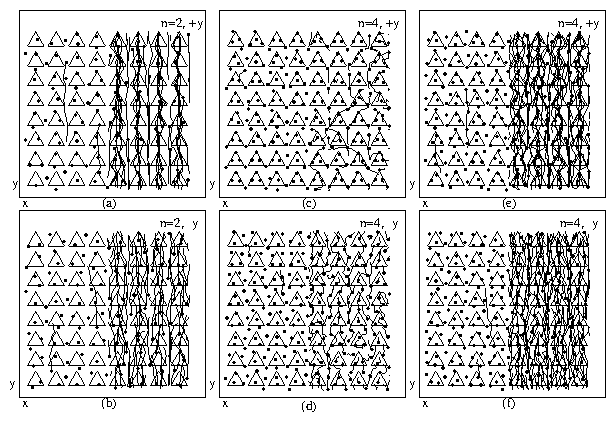
 Figure 3: Pins (triangles), vortices (dots), and vortex
trajectories (lines for all vortices in the right half of the panels,
and for two selected vortices in the left half of the panels)
during 1/10 of the driving period for: (a)
N=2, A=1.0, +y portion of drive cycle, (b) −y
portion of same cycle; (c) N=4, A=0.35, +y portion
of drive cycle, (d) −y portion of same cycle; (e) N=4,
A=0.75, +y portion of drive cycle, (f) −y
portion of same cycle.
Figure 3: Pins (triangles), vortices (dots), and vortex
trajectories (lines for all vortices in the right half of the panels,
and for two selected vortices in the left half of the panels)
during 1/10 of the driving period for: (a)
N=2, A=1.0, +y portion of drive cycle, (b) −y
portion of same cycle; (c) N=4, A=0.35, +y portion
of drive cycle, (d) −y portion of same cycle; (e) N=4,
A=0.75, +y portion of drive cycle, (f) −y
portion of same cycle.
|