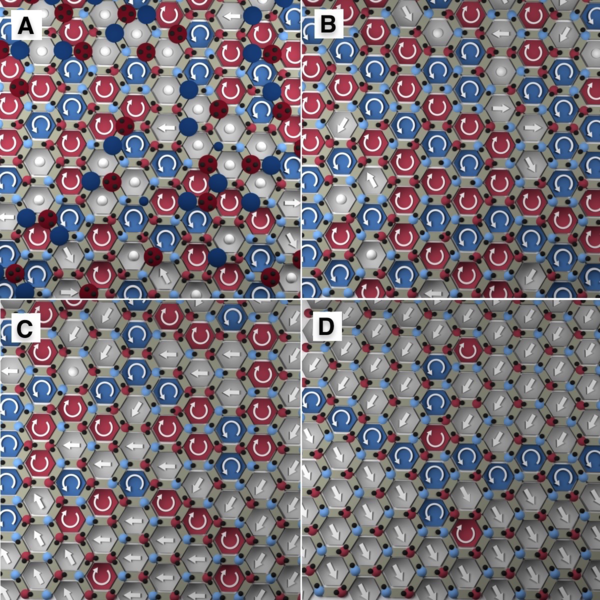
 Figure 1: Schematic of the particle-based
hexagonal artificial spin ice.
Each double well trap (light grey) holds a single
paramagnetic colloid (dark grey dots).
The hexagonal plaquettes contain arrows indicating
the pseudospin →σi of the adjacent traps, colored according to
the chirality
χi=+1 (clockwise, dark grey) or χi=−1 (counter-clockwise, white).
The plaquettes are colored according to their net spin chirality χ:
clockwise (red), counter-clockwise (blue), or achiral (grey).
Colored disks are guides to the eye and indicate the vertex type:
n=0 or 0-in (dark blue), n=1 (light blue), n=2 (light red), and
n=3 (dark red); arrows (of length 2) or dots (of length 0) on the
disks indicate the vectorial sum →si of the pseudospins adjacent to each vertex.
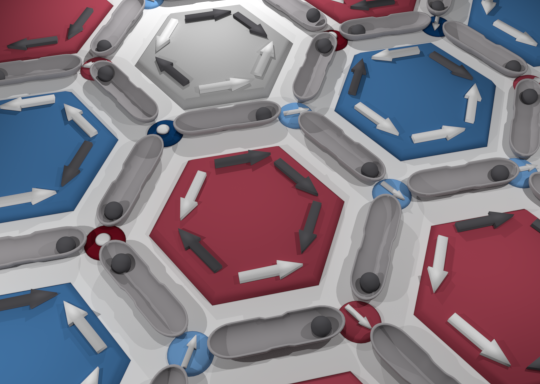
Figure 1: Schematic of the particle-based
hexagonal artificial spin ice.
Each double well trap (light grey) holds a single
paramagnetic colloid (dark grey dots).
The hexagonal plaquettes contain arrows indicating
the pseudospin →σi of the adjacent traps, colored according to
the chirality
χi=+1 (clockwise, dark grey) or χi=−1 (counter-clockwise, white).
The plaquettes are colored according to their net spin chirality χ:
clockwise (red), counter-clockwise (blue), or achiral (grey).
Colored disks are guides to the eye and indicate the vertex type:
n=0 or 0-in (dark blue), n=1 (light blue), n=2 (light red), and
n=3 (dark red); arrows (of length 2) or dots (of length 0) on the
disks indicate the vectorial sum →si of the pseudospins adjacent to each vertex.
|