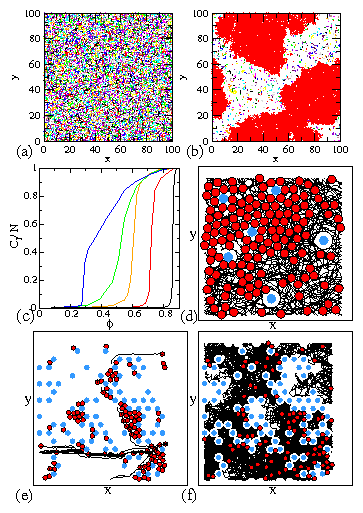
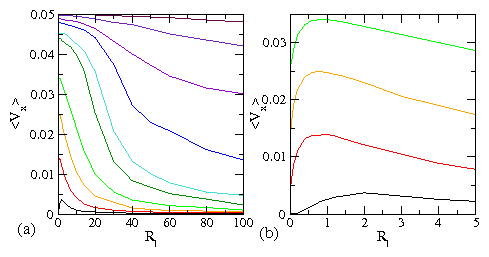
 Figure 2:
(a)
〈Vx〉, the average velocity per particle in the drift direction,
vs Rl
for a system with
ϕ = 0.667
and
obstacle density
ϕp = 0.00039, 0.00157, 0.00472, 0.0785, 0.0157, 0.02356, 0.055,
0.0942, 0.01413, and 0.188, from top to bottom.
When Rl > 5.0, 〈Vx〉
decreases with increasing Rl.
(b)
〈Vx〉 vs Rl passes through a maximum for all the
curves, as shown more clearly in this blow-up of panel (a) for
ϕp = 0.055, 0.094, 0.1413, and 0.188, from top to bottom.
Figure 2:
(a)
〈Vx〉, the average velocity per particle in the drift direction,
vs Rl
for a system with
ϕ = 0.667
and
obstacle density
ϕp = 0.00039, 0.00157, 0.00472, 0.0785, 0.0157, 0.02356, 0.055,
0.0942, 0.01413, and 0.188, from top to bottom.
When Rl > 5.0, 〈Vx〉
decreases with increasing Rl.
(b)
〈Vx〉 vs Rl passes through a maximum for all the
curves, as shown more clearly in this blow-up of panel (a) for
ϕp = 0.055, 0.094, 0.1413, and 0.188, from top to bottom.
|