Crossover from Jamming to Clogging Behaviours in Heterogeneous Environments

H. Péter ^1,2, A. Libál ^1,2, C. Reichhardt ¹, C.J.O. Reichhardt ¹

¹Theoretical Division and Center for Nonlinear Studies, Los Alamos National Laboratory, Los Alamos, New Mexico, 87545, USA. ² Faculty of Mathematics and Computer Science, Babes-Bolyai University, Cluj, 400084, Romania.

Jamming describes a transition from a flowing or liquid state to a solid or rigid state in a loose assembly of particles such as grains or bubbles. In contrast, clogging describes the ceasing of the flow of particulate matter through a bottleneck. It is not clear how to distinguish jamming from clogging, nor is it known whether they are distinct phenomena or fundamentally the same. We examine an assembly of disks moving through a random obstacle array and identify a transition from clogging to jamming behavior as the disk density increases. The clogging transition has characteristics of an absorbing phase transition, with the disks evolving into a heterogeneous phase-separated clogged state after a critical diverging transient time. In contrast, jamming is a rapid process in which the disks form a homogeneous motionless packing, with a rigidity length scale that diverges as the jamming density is approached.

---

Results
Time evolution to a jammed or clogged state
Velocity measurement of the transition from clogging to jamming
Transient velocities near the clogging and jamming transitions
Local disk densities in clogged and jammed states
Discussion
Methods
References

---

The concept of jamming is used in loose assemblies of particles such as grains or bubbles to describe the transition from an easily flowing fluidlike state to a rigid jammed or solidlike state [1,2,3,4]. Liu and Nagel proposed a generalized jamming phase diagram combining temperature, load, and density, where a particularly important point is the density ϕ_j at which jamming occurs [1]. Jamming has been extensively studied in a variety of systems [3,4,5], and there is evidence that in certain cases, the jamming transition has the properties of a critical point, such as a correlation length that diverges as the jamming density is approached [2,3,4,5,6,7,8,9]. A related phenomenon is the clogging that occurs for particles flowing through a hopper, where as a function of time there is a probability for arch structures to form that block the flow [10,11,12,13]. Clogging is associated with the motion of particulate matter past a physical constraint such as wells, barriers, obstacles, or bottlenecks [14,15,16,17,18,19]; however, it has not been established whether jamming and clogging are two forms of the same phenomenon or whether there are key features that distinguish jamming from clogging.

Here we show for frictionless disks moving through a random obstacle array that jamming and clogging are distinct phenomena and that a transition from clogging to jamming occurs as a function of increasing disk density. We identify the number of obstacles required to stop the flow and the transient times needed to reach a stationary clogged or jammed state. There are two critical obstacle densities, ϕ_c^j for the jammed state and ϕ_c^c for the clogged state. In the jamming regime, the obstacle density ϕ_c^j at which flow ceases drops to lower obstacle densities with increasing disk density, and the system forms a homogeneous jammed state when the rigidity correlation length associated with ϕ_j becomes larger than the average distance between obstacles. In contrast, during clogging the system organizes over time into a heterogeneous or phase-separated state, and the transient time diverges at a critical obstacle density ϕ_c^c that is independent of the disk density. The phase-separated state consists of regions with a density near ϕ_j coexisting with low density regions.

Results

Figure 1: Figure 1: Clogging in obstacle arrays. Images of (a, d) initial, (b, e) transient flowing, and (c, f) final clogged state for mobile disks (dark blue open circles) driven in the positive x direction through obstacles (red filled circles) in a sample with obstacle density ϕps=0.175 and disk density (a, b, c) ϕp=0.2186 and (d, e, f) ϕp=0.436. Green lines in (b,e) indicate the disk trajectories over a fixed time period. The disks are initially in a flowing state and evolve into a phase-separated clogged state. The dense regions have a local disk density of ϕloc=0.84.

Figure 2: Jamming in obstacle arrays under increasing obstacle density in a sample with ϕp=0.785. The randomly placed obstacles prevent the monodisperse disks from forming a state with crystalline ordering. (a, c, e) Mobile disks (dark blue open circles) and obstacles (red filled circles). (b, d, f) Corresponding disk trajectories over a fixed time period (green lines) and obstacle locations (red filled circles), with the mobile disks omitted for clarity. (a, b) At an obstacle density of ϕps = 0.043, we find steady state flow. (c, d) At ϕps=0.065 the steady state flow is reduced but still present. (e, f) At ϕps = 0.0872, the system jams. The jammed state is much more uniform in density than the clogged state, and jamming occurs rapidly with almost no transient flow above a critical obstacle density ϕcj.

Time evolution to a jammed or clogged state

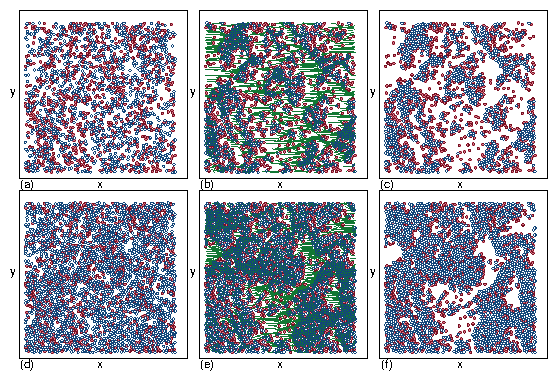
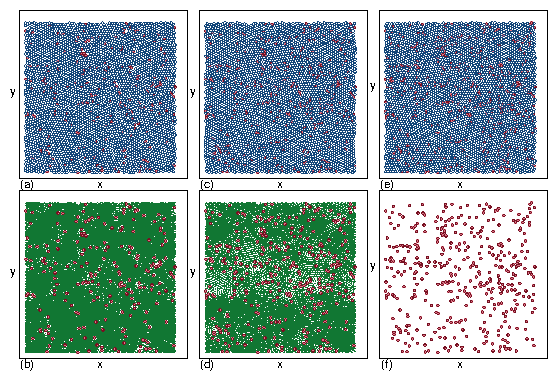
We numerically examine disks driven through a two-dimensional array of obstacles in the form of immobile disks. Simulation details appear in the Methods section. The total area density of the system is ϕ_tot=ϕ_p + ϕ_ps, where ϕ_p is the area density of the moving disks and ϕ_ps is the area density of the obstacles. Starting from a uniformly dense sample, we apply a driving force and find that over time the system evolves either to a steady free flowing state or to a motionless clogged or jammed state. In Fig. 1a,b,c we illustrate the time evolution of a system with a disk density of ϕ_p = 0.2186 and an obstacle density of ϕ_ps = 0.175, beginning with the uniform density initial state in Fig. 1a. Upon application of a drive, we find a transient flowing state as shown in Fig. 1b which gradually evolves into the final motionless phase-separated or clustered clogged state in Fig. 1c. For a higher disk density of ϕ_p=0.436, Fig. 1d,e,f shows that the same evolution from uniform initial state to transient flowing state to static clogged state occurs, but the dense clusters in the clogged state are larger. At much higher disk densities of ϕ_p=0.785, we find jamming behavior when the obstacle density is larger than a critical value ϕ_c^j. Below ϕ_c^j, the system quickly settles into steady state flow, as shown in Fig. 2(a,b) for ϕ_ps=0.043 and in Fig. 2(c,d) for ϕ_ps=0.065. The magnitude of the flow decreases with increasing ϕ_ps. Above ϕ_c^j the disks quickly form a disordered jammed state when driven, as illustrated in Fig. 2(e,f) for ϕ_ps=0.0872. In contrast to the density phase-separated clogged states that form at lower ϕ_p, the jammed states are homogeneously dense. The randomly placed obstacles prevent the monodisperse disks from developing long-range crystalline order.

Figure 3: Clogging-jamming phase diagram. The heat map of the disk velocity V0 after 106 simulation time steps as a function of obstacle density ϕps vs disk density ϕp. Yellow indicates high V0 and blue indicates zero V0. The white region at the upper right is above the density ϕtot = π/2√3 ≈ 0.9069 at which the disks would form a hexagonal solid, and thus lies outside the range of our model. Clogging occurs for ϕp < 0.67, and the critical obstacle density for clogging ϕcc ≈ 0.15, is nearly independent of ϕp. Jamming occurs for ϕp > 0.67, as indicated by the red vertical dashed line, and ϕcj, the critical obstacle density for jamming, decreases linearly with increasing ϕp, ranging from 0.15 > ϕcj > 0. The dots along ϕp=0.234 indicate the values of ϕps shown in the time series of Fig. 4a, while the dots along ϕp=0.785 indicate the values of ϕps shown in the time series of Fig. 4b.

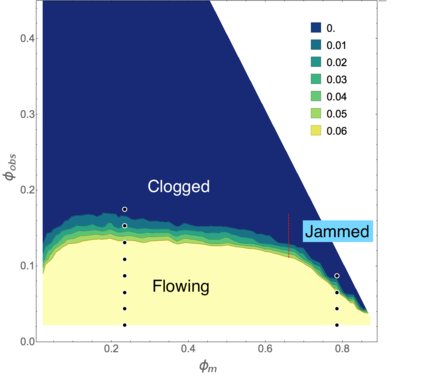
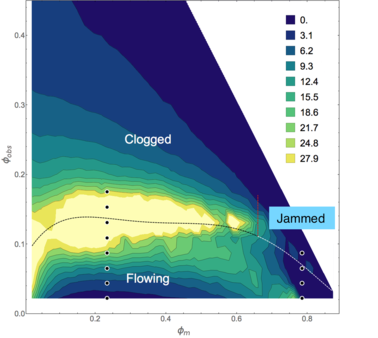
Velocity measurement of the transition from clogging to jamming

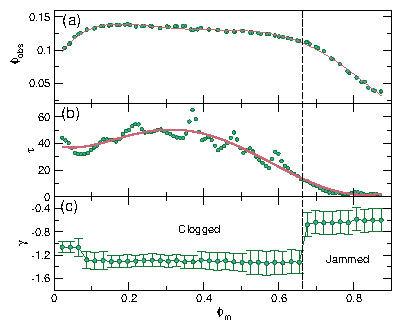
To characterize the system we perform a series of simulations with varied ϕ_p and ϕ_ps. We measure the final velocity V₀ of the mobile disks after a fixed time interval, and average over ten different realizations. In Fig. 3 we plot a velocity heat map as a function of ϕ_ps versus ϕ_p. We find a flowing regime at small ϕ_ps, a clogged regime for ϕ_p < 0.67, and a jammed regime for ϕ_p > 0.67. The critical obstacle density ϕ_c^c above which the velocity V₀ drops to zero in the clogging regime remains roughly constant at ϕ_c^c ≈ 0.15, independent of the value of ϕ_p. This indicates that the transition to a clogged state is controlled by the average spacing l_ps=1/√{ϕ_ps} between obstacles, similar to the manner in which hopper clogging is controlled by the aperture size. In contrast, in the jamming regime the critical obstacle density ϕ_c^j separating flowing from jammed states decreases linearly with increasing ϕ_p and reaches ϕ_c^j=0 for ϕ_p ≈ 0.9069, indicating that this transition is controlled by a growing correlation length ξ associated with the jamming point ϕ_j [5]. We argue that the system jams when ξ = l_ps. If we assume that near jamming in a clean system, the correlation length grows as ξ ∝ (ϕ_j − ϕ_p)^−ν, then the transition to the jammed state varies with obstacle density according to ϕ_c^j ∝ (ϕ_j −ϕ_p)^2ν. In Fig. 3, ϕ_c^j ∝ ϕ_p, implying that ν = 1/2, consistent with the exponent ν = 1/2 proposed for jamming in Refs. [20,21], as well as with simulation measurements giving ν in the range 0.6 to 0.7 for two-dimensional bidisperse disks [6,8]. The exponent we find is also in agreement with that observed for the shift in the jamming point in bidisperse disks on random pinning arrays [22]. Studies of bidisperse disk jamming with dilute obstacles very near ϕ_j also show that ϕ_j decreases linearly with obstacle density, giving ν = 1/2 [23].

Previous simulations of bidisperse disks of radius R_s=0.5 and R_l=0.7 flowing through a periodic array of obstacles with radius R_s=0.5 showed that clogging is strongly enhanced when l_ps <~2.35 [24]. This is because in order for a pair of disks, one large and one small, to fit between two obstacles, the lattice constant a of the obstacle array must be at least large enough to accommodate the size of the obstacle itself plus the size of the two disks, a ≥ 2R_s+2R_s+2R_l=2.4. In our monodisperse disk system, the obstacles are placed randomly, but one can obtain an estimate of the l_ps for the onset of clogging by considering the circular holes in the obstacle array [25]. If we construct a circle that just touches any three disks and/or obstacles, this circle is defined to be a hole when it does not overlap any disks. For a pair of disks to pass between two obstacles, the obstacle spacing must once again accommodate the size of the obstacle itself plus the size of the two disks, l_ps ≥ l_ps^c = 2R_d+2R_d+2R_d=3.0. This spacing can be achieved by placing the obstacles such that circular holes of size R_d can form on all sides of the hole on average, giving an effective obstacle radius of 3R_d and a critical obstacle density of ϕ_ps^c=(1/6)(π/(2√3))=0.15. Below ϕ_ps^c, the holes percolate and the disks can flow, while above ϕ_ps^c, not enough holes are available to permit steady state free flow and a clogged state forms. In Fig. 3, the onset of clogging, ϕ_c^c ≈ 0.15, is close to ϕ_ps^c. When ϕ_p <~0.15, ϕ_c^c is no longer constant but decreases with decreasing ϕ_p. At these low disk densities, mobile disks are trapped independently, so at least one additional obstacle must be added for every additional mobile disk, giving ϕ_c^c ∝ ϕ_p.

Figure 4: Transient times for clogging and jamming. (a) The average velocity V per mobile disk vs time in simulation time steps for samples with mobile disk density ϕp = 0.234 at varied obstacle density ϕps=0.087, 0.109, 0.131, 0.153, and 0.175, from top to bottom. Dashed lines indicate fits to V ∝ Aexp(−t/τ) +V0. The disks reach a clogged state for ϕps > 0.15. (b) V vs time for samples with ϕp=0.785 in the jamming regime for ϕps=0.022, 0.044, 0.065, and 0.087, from top to bottom. The transient times are very short.

Transient velocities near the clogging and jamming transitions

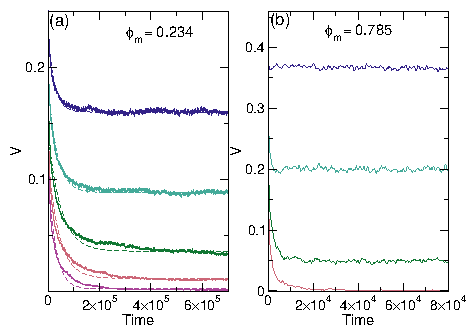
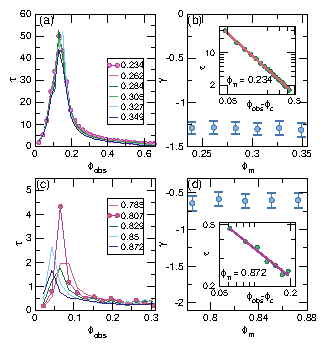
In Fig. 4a we show representative time series of the average velocity V per mobile disk in the clogging regime at ϕ_p = 0.234 for ϕ_ps ranging from ϕ_ps=0.087 to 0.175. At low obstacle densities, the disks reach a steady state flow after a very short transient time τ. As ϕ_ps increases, τ increases, showing a divergence at the critical obstacle density ϕ_c^c where clogging first occurs, while for ϕ_ps > ϕ_c^c, τ decreases with increasing ϕ_ps and the disks reach a completely clogged state with V=0. We fit V(t) ∝ Aexp(−t/τ) + V₀, as indicated by the dashed lines in Fig. 4(a), and plot the resulting values of τ in Fig. 5a as a function of ϕ_ps for ϕ_p=0.234 to 0.349. In each case, τ diverges near ϕ_ps = 0.15. We fit this divergence for ϕ_ps > ϕ_c^c to a power law, τ ∝ (ϕ_ps − ϕ_c^c)^γ, as shown in the inset of Fig. 5b for ϕ_p = 0.234, where γ = −1.29 ±0.1. The plot of γ versus ϕ_p in the main panel of Fig. 5(b) indicates that γ has a constant value in the range −1.25 to −1.35. The transient time behavior is similar to that found for the diverging time scales that appear near the irreversible-reversible transition in systems exhibiting random organization [26,27,28] and near the depinning transition for colloids [29] and vortices [30,31] driven over random pinning arrays. The power law exponents are also close to the value γ = −1.295 expected for the universality class of two-dimensional directed percolation [32], and we find similar values of γ for ϕ_p < 0.67 throughout the clogging regime. Directed percolation is often used to describe nonequilibrium absorbing phase transitions [32], and in our case the steady state flow corresponds to a fluctuating state, while the clogged state is the non-fluctuating or absorbed state.

Figure 5: Transient times for clogging and jamming. (a) Transient times τ vs ϕps obtained from V(t) curves such as those shown in Fig. 4a by fitting V ∝ Aexp(−t/τ) +V0 for ϕp=0.234 to 0.349, from top to bottom. There is a divergence in τ near the clogging density of ϕcc = 0.15. (b) The value of the exponent γ vs ϕp obtained by fitting the curves in a to τ ∝ (ϕps − ϕcc)γ. Inset: τ vs ϕps−ϕcc at ϕp=0.234. The pink line indicates a power law fit with γ = −1.29 ±0.1. (c) τ vs ϕps obtained from the V(t) curves such as those shown in Fig. 4b for ϕp=0.785 to 0.872, from top to bottom. The transient times are much shorter than those in the clogging regime in panel a. (d) Exponent γ vs ϕp obtained by fitting the curves in c to τ ∝ (ϕps − ϕcj)γ. Inset: τ vs ϕps−ϕcj at ϕp=0.872. The pink line indicates a power law fit with γ = −0.66.

In the jamming regime the transient times are much shorter, as shown by the plot of V(t) in Fig. 4b for ϕ_p=0.785 at ϕ_ps=0.022 to 0.087. In Fig. 5c, τ versus ϕ_ps in the range ϕ_p=0.785 to 0.872 has a value that is an average of 20 times smaller than in the clogging regime from Fig. 5a. The peak in τ shifts to lower ϕ_ps with increasing ϕ_p, reflecting the behavior of the critical jamming density ϕ_c^j. By fitting the curves in Fig. 5c to τ ∝ (ϕ_ps−ϕ_c^j)^γ, as demonstrated in the inset of Fig. 5d for ϕ_p=0.872, we obtain γ ≈ −0.66, as shown in the plot of γ versus ϕ_p in the main panel of Fig. 5d. This indicates that there is a pronounced difference in the dynamics of the jamming regime compared to the clogging regime.

Figure 6: Transient time behavior. The heat map of the transient times τ obtained from fitting V(t)=Aexp(−t/τ) + V0 as a function of ϕps vs ϕp. Yellow indicates large τ and blue indicates small τ. The dark dashed line is a guide to the eye marking the crossover from a flowing state to a clogged state, while the white dashed line indicates the transition from a flowing state to a jammed state. The white region is above the maximum density ϕtot=π/2√3 of our model. The dots along ϕp = 0.234 indicate the values of ϕps shown in the time series of Fig. 4a, while the dots along ϕp=0.785 indicate the values of ϕps shown in the time series of Fig. 4b. The system must organize into a clogged state, giving large transient times in the clogging regime, but can quickly enter a jammed state, giving small transient times in the jamming regime.

In Fig. 6 we show a heat map of the transient time τ obtained by fitting V(t)=Aexp(−t/τ) + V₀. The transient times become large near the crossover from flowing to clogging for ϕ_p < 0.67, while in the jamming regime for ϕ_p > 0.67, the transient times are strongly reduced. This provides further evidence that in the clogging regime it is necessary for the system to organize over time into a clogged state, gradually forming phase-separated regions of high and low density as illustrated in Fig. 1. In contrast, the jammed system has strong spatial correlations, and once the correlation length associated with ϕ_j is larger than the distance l_ps between defects, very few disk rearrangements are needed to bring the system into a stationary, nonflowing state.

Figure 7: Transient times and critical exponents across the clogging to jamming transition. (a) The location of the transition from a flowing state to a clogged or jammed state, defined as points for which V0=0.01, as a function of ϕps vs ϕp. The dashed line separates clogged states at low ϕp from jammed states at high ϕp. (b) The transient time τ at the flowing to nonflowing transition point vs ϕp. (c) The transient exponent γ extracted from the nonflowing side of the transition vs ϕp. There is a clear crossover from clogging to jamming. In the clogging regime, γ ≈ −1.29, but in the jamming regime, γ ≈ −0.66, indicating that the dynamics of clogging differ from those of jamming.

In Fig. 7a we show the transition from the flowing to the clogged or jammed state as a function of ϕ_ps versus ϕ_p by identifying the points from Fig. 3 for which V=0.01. Figure 7b shows the transient times τ along this transition line, and in Fig. 7c we plot the transient exponent γ. The dashed vertical line at ϕ_p=0.67 indicates a transition from clogging to jamming behavior, correlated with a change from γ ≈ −1.29 in the clogging regime to γ ≈ −0.66 in the jamming regime, as well as with a drop in ϕ_ps and τ. The point ϕ_p = 0.67 matches the density at which 2D continuum percolation of disks is expected to occur. We find a third value of γ for ϕ_p < 0.07 in a density regime where the value of ϕ_ps at which a clogged state appears decreases with decreasing ϕ_p. This regime is dominated by the trapping of single disks rather than collective clogging dynamics.

Figure 8: Local density distributions in clogged and jammed systems. (a) The local density distribution P(ϕloc) averaged over 10 realizations for a system with ϕp = 0.5 and ϕps = 0.175 that reaches a clogged state in which the local density is bimodally distributed. Inset: Image of a clogged configuration from one of the realizations, showing the mobile disks (dark blue open circles) trapped by the obstacles (red filled circles). (b) P(ϕloc) averaged over ten realizations for a jammed system with ϕp = 0.8 and ϕps = 0.06 shows a single peak at ϕtot. Inset: Image of a jammed disordered configuration from one of the realizations.

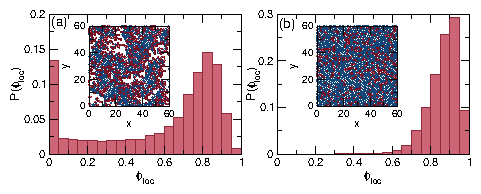
Local disk densities in clogged and jammed states.

The clogged and jammed systems can also be distinguished by examining the local disk density ϕ_loc measured in areas 6R_d ×6R_d in size. In Fig. 8a we plot the local density distribution P(ϕ_loc) averaged over ten realizations of the final clogged state for a system with ϕ_p = 0.5 and ϕ_ps = 0.175. As shown in the inset of Fig. 8a, the disks phase separate into low density regions associated with the peak at ϕ_loc=0.1 and high density regions which produce a second peak at ϕ_loc=0.85. The local density of the dense regions is lower than the value of ϕ_loc=0.9069 for a dense ordered hexagonal disk arrangement due to the considerable disorder introduced in the packing by the randomly placed obstacles. In Fig. 8b, P(ϕ_loc) for a system with ϕ_p = 0.8 and ϕ_ps = 0.06 that reaches a jammed state has a single peak near ϕ_loc=0.9, reflecting the uniform disk density at jamming that is illustrated in the inset of Fig. 8(b).

Discussion

Our results suggest that clogging and jamming processes have different dynamics. Clogging in the presence of random obstacles has signatures of an absorbing transition falling in a directed percolation universality class, and its dynamics are controlled by the average spacing of the obstacles. In the jamming that occurs for higher ϕ_tot, the dynamics are controlled by the growing correlation length associated with ϕ_j, the jamming density of an obstacle-free system. These results show that jamming and clogging in obstacles are indeed different phenomena. Jamming is associated with an equilibrium critical point, the formation of a homogeneous rigid state, and short transient times to reach this state, while clogging is a nonequilibrium dynamical phenomenon in which the system evolves over an extended time into a strongly spatially heterogeneous state. Our results have implications for flow though heterogeneous media [33], erosion [34], depinning transitions in particle assemblies [35], and active matter in disordered environments [36,37]. Experimentally our results could be tested using colloidal particles at low flow rates to reduce hydrodynamic effects. It would also be interesting to examine the effects of adding frictional contacts between the disks, since these can change the characteristics of the jamming transition [38,39], or to replace the disks by elongated particles [40] or chains [41,42].

Methods

Numerical simulation details

We conduct simulations of nonoverlapping disks and obstacles confined to a two-dimensional plane. The system size is L ×L with L=60, and we use periodic boundary conditions in both the x and y directions. We introduce N_p mobile disks of radius R_d=0.5 along with N_ps obstacles represented by disks of radius R_d that are not allowed to move. The area coverage of the mobile disks is ϕ_p=N_pπR_d²/L², the area coverage of the obstacles is ϕ_ps=N_psπR_d²/L², and the total area coverage is ϕ_tot=ϕ_p+ϕ_ps.

The disk dynamics are given by the overdamped equation of motion

1 η ∆ri ∆t = Fppi + Fobsi + Fd

(1)

where η = 1 is the viscosity. The interaction between two disks at r_i and r_j is a short range harmonic repulsion, F_dd^ij=k(r_ij−2R_d)Θ(r_ij−2R_d)∧r_ij, where r_ij=|r_i−r_j|, ∧r_ij=(r_i−r_j)/r_ij, and Θ is the Heaviside step function. We set k = 200, which is large enough that overlap between disks does not exceed 0.01R_d, placing us in the hard disk limit. The interactions with mobile disks are given by F_ppⁱ=∑_{j ≠ i}^N_pF_dd^ij, while the interactions with obstacles are given by F_obsⁱ=∑_j^N_psF_dd^ij. We apply a uniform driving force F_d=F_d∧x to all mobile disks, with F_d=0.5. Distances are measured in simulation units l₀ and forces are measured in simulation units f₀ so that k is in units of f₀/l₀ and the unit of simulation time is t₀=ηl₀/f₀. We initialize the system by placing N_p+N_ps disks of reduced radius in randomly chosen nonoverlapping positions, and then gradually expanding the radii to size R_d while allowing all disks to move. This produces a randomized packing of homogeneous density with no internal tensions. We then randomly assign N_ps of the disks to be obstacles, and apply an external driving force. After a fixed simulation time of 1 ×10⁶ simulation time steps, we determine whether the system has reached a clogged or jammed state based on whether the average disk velocity V has dropped to zero.

References

[1]

Liu, A. J. & Nagel, S.R. Nonlinear dynamics: Jamming is not just cool any more. Nature (London) 396, 21-22 (1998).

[2]

O'Hern, C. S., Silbert, L. E., Liu, A. J. & Nagel, S. R. Jamming at zero temperature and zero applied stress: The epitome of disorder. Phys. Rev. E 68 011306 (2003).

[3]

van Hecke, M. Jamming of soft particles: geometry, mechanics, scaling and isostaticity. J. Phys. Condens. Matter 22, 033101 (2010).

[4]

Liu, A. J. & Nagel, S. R. The jamming transition and the marginally jammed solid. Annu. Rev. Condens. Matter Phys. 1, 347-369 (2010).

[5]

Reichhardt, C. & Olson, C. J. Aspects of jamming in two-dimensional athermal frictionless systems. Soft Matter 10, 2932-2944 (2014).

[6]

Drocco, J. A., Hastings, M. B., Reichhardt, C. J. O. & Reichhardt, C. Multiscaling at Point J: Jamming is a critical phenomenon. Phys. Rev. Lett. 95, 088001 (2005).

[7]

Henkes, S. & Chakraborty, B. Jamming as a critical phenomenon: A field theory of zero-temperature grain packings. Phys. Rev. Lett. 95, 198002 (2005).

[8]

Olsson, P. & Teitel, S. Critical scaling of shear viscosity at the jamming transition. Phys. Rev. Lett. 99, 178001 (2007).

[9]

Candelier, R. & Dauchot, O. Creep motion of an intruder within a granular glass close to jamming. Phys. Rev. Lett. 103, 128001 (2009).

[10]

To, K., Lai, P.-Y. & Pak, H. K. Jamming of granular flow in a two-dimensional hopper. Phys. Rev. Lett. 86, 71-74 (2001).

[11]

Zuriguel, I., Pugnaloni, L. A., Garcimartín, A. & Maza, D. Jamming during the discharge of grains from a silo described as a percolating transition. Phys. Rev. E 68, 030301(R) (2003).

[12]

Chen, D., Desmond, K. W. & Weeks, E. R. Topological rearrangements and stress fluctuations in quasi-two-dimensional hopper flow of emulsions. Soft Matter 8, 10486-10492 (2012).

[13]

Thomas, C. C. & Durian, D. J. Geometry dependence of the clogging transition in tilted hoppers. Phys. Rev. E 87, 052201 (2013).

[14]

Redner, S. & Datta, S. Clogging time of a filter. Phys. Rev. Lett. 84, 6018-6021 (2000).

[15]

Wyss, H. M., Blair, D. L., Morris, J. F., Stone, H. A. & Weitz, D. A. Mechanism for clogging of microchannels. Phys. Rev. E 74, 061402 (2006).

[16]

Roussel, N., Nguyen, T. L. H. & Coussot, P. General probabilistic approach to the filtration process. Phys. Rev. Lett. 98, 114502 (2007).

[17]

Zuriguel, I. et al. Clogging transition of many-particle systems flowing through bottlenecks. Sci. Rep. 4, 7324 (2014).

[18]

Agbangla, G. C., Bacchin, P. & Climent, E. Collective dynamics of flowing colloids during pore clogging. Soft Matter 10, 6303-6315 (2014).

[19]

Dressaire, E. & Sauret, A. Clogging of microfluidic systems. Soft Matter 13, 37-48 (2017).

[20]

Wyart, M., Nagel, S. R. & Witten, T. A. Geometric origin of excess low-frequency vibrational modes in weakly connected amorphous solids. Europhys. Lett. 72, 486-492 (2005).

[21]

Wyart, M., Silbert, L. E., Nagel, S. R. & Witten, T. A. Effects of compression on the vibrational modes of marginally jammed solids. Phys. Rev. E 72, 051306 (2005).

[22]

Reichhardt, C. J. O., Groopman, E., Nussinov, Z. & Reichhardt, C. Jamming in systems with quenched disorder. Phys. Rev. E 86, 061301 (2012).

[23]

Graves, A. L., Nashed, S., Padgett, E., Goodrich, C. P, Liu, A. J. & Sethna, J. P. Pinning susceptibility: The effect of dilute, quenched disorder on jamming. Phys. Rev. Lett. 116, 235501 (2016).

[24]

Nguyen, H. T., Reichhardt, C. & Reichhardt, C. J. O. Clogging and jamming transitions in periodic obstacle arrays. Phys. Rev. E 95, 030902 (2017).

[25]

Hinrichsen, E. L., Feder, J. & Jøssang, T. Geometry of random sequential adsorption. J. Stat. Phys. 44, 793-827 (1986).

[26]

Corte, L., Chaikin, P. M., Gollub, J. P. & Pine, D. J. Random organization in periodically driven systems. Nature Phys. 4, 420-424 (2008).

[27]

Milz, L. & Schmiedeberg, M. Connecting the random organization transition and jamming within a unifying model system. Phys. Rev. E 88, 062308 (2013).

[28]

Tjhung, E. & Berthier, L. Criticality and correlated dynamics at the irreversibility transition in periodically driven colloidal suspensions. J. Stat. Mech. 2016 033501 (2016).

[29]

Reichhardt, C. & Reichhardt, C. J. O. Random organization and plastic depinning. Phys. Rev. Lett. 103, 168301 (2009).

[30]

Shaw, G., Mandal, P., Banerjee, S. S., Niazi, A., Rastogi, A. K., Sood, A. K., Ramakrishnan, S. & Grover, A. K. Critical behavior at depinning of driven disordered vortex matter in 2H-NbS₂. Phys. Rev. B 85, 174517 (2012).

[31]

Okuma, S., Tsugawa, Y. & Motohashi, A. Transition from reversible to irreversible flow: Absorbing and depinning transitions in a sheared-vortex system. Phys. Rev. B 83, 012503 (2011).

[32]

Hinrichsen, H. Non-equilibrium critical phenomena and phase transitions into absorbing states. Adv. Phys. 49, 815-958 (2000).

[33]

Mays, D. C. & Hunt, J. R. Hydrodynamic aspects of particle clogging in porous media. Environ. Sci. Technol. 39, 577-584 (2005).

[34]

Aussillous, P., Zou, Z., Guazzelli, E., Yan, L. & Wyart, M. Scale-free channeling patterns near the onset of erosion of sheared granular beds. Proc. Natl. Acad. Sci. 113, 11788-11793 (2016).

[35]

Reichhardt, C. & Reichhardt, C. J. O. Depinning and nonequilibrium dynamic phases of particle assemblies driven over random and ordered substrates: a review. Rep. Prog. Phys 80, 026501 (2017).

[36]

Bechinger C., Di Leonardo R., Löwen, H., Reichhardt, C., Volpe, G. & Volpe, G. Active particles in complex and crowded environments. Rev. Mod. Phys. 88, 045006 (2016).

[37]

Morin, A., Desreumaux, N., Caussin, J.-B. & Bartolo, D. Distortion and destruction of colloidal flocks in disordered environments. Nature Phys. 13, 63-67 (2017).

[38]

Bi, D., Zhang, J., Chakraborty, B. & Behringer, R. P. Jamming by shear. Nature 480, 335 (2011).

[39]

Henkes, S., Quint, D. A., Fily, Y. & Schwarz, J. M. Rigid cluster decomposition reveals criticality in frictional jamming. Phys. Rev. Lett. 116, 028301 (2016).

[40]

Gerbode, S. J., Agarwal, U., Ong, D. C., Liddell, C. M., Escobedo, F. & Cohen, I. Glassy dislocation dynamics in 2D colloidal dimer crystals. Phys. Rev. Lett. 105, 078301 (2010).

[41]

Zou, L.-N., Cheng, X., Rivers, M. L., Jaeger, H. M. & Nagel, S. R. The packing of granular polymer chains. Science 326, 408-410 (2009).

[42]

Lopatina, L. M., Reichhardt, C. J. O. & Reichhardt, C. Jamming in granular polymers. Phys. Rev. E 84, 011303 (2011).

Acknowledgments

This work was carried out under the auspices of the National Nuclear Security Administration of the U.S. Department of Energy at Los Alamos National Laboratory under Contract No. DEAC52-006NA25396. The authors wish to thank LDRD at LANL for financial support through grant 20170147ER.

Author contributions:

C.R. and C.J.O.R. conceived the work and wrote the manuscript, H.P. and A.L. conducted the simulations and performed data analysis.

Additional information

Competing interests: The authors declare no competing interests.

---

File translated from T_EX by T_THgold, version 4.00.
Back to Home