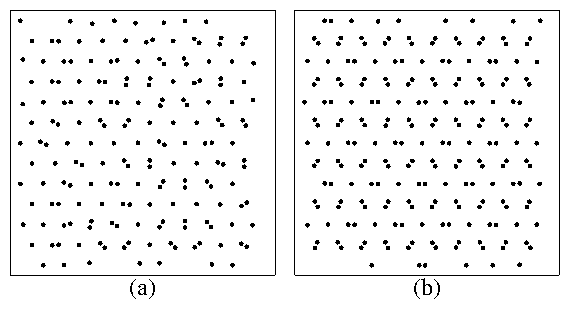
 Figure 3:
The colloidal positions (black dots) and trajectories (lines) over a
fixed period of time
for A = 3.0 and a filling of Nc/Ns=9/4 at different
temperatures.
(a) Phase I, trimer-dimer solid, at T/Tm = 0.
(b) Phase II, trimer liquid, dimer solid phase, at T/Tm = 0.56.
(c) Phase III, trimer-dimer liquid, at T/Tm = 2.4. (d)
Modulated liquid phase, at T/Tm = 5.0.
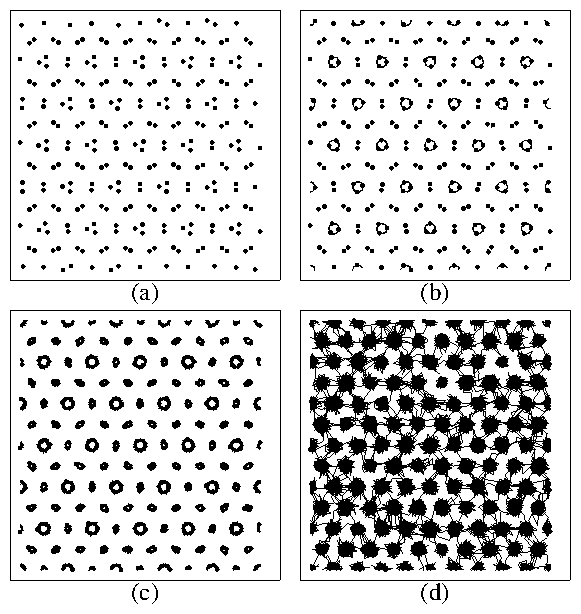
Figure 3:
The colloidal positions (black dots) and trajectories (lines) over a
fixed period of time
for A = 3.0 and a filling of Nc/Ns=9/4 at different
temperatures.
(a) Phase I, trimer-dimer solid, at T/Tm = 0.
(b) Phase II, trimer liquid, dimer solid phase, at T/Tm = 0.56.
(c) Phase III, trimer-dimer liquid, at T/Tm = 2.4. (d)
Modulated liquid phase, at T/Tm = 5.0.
|