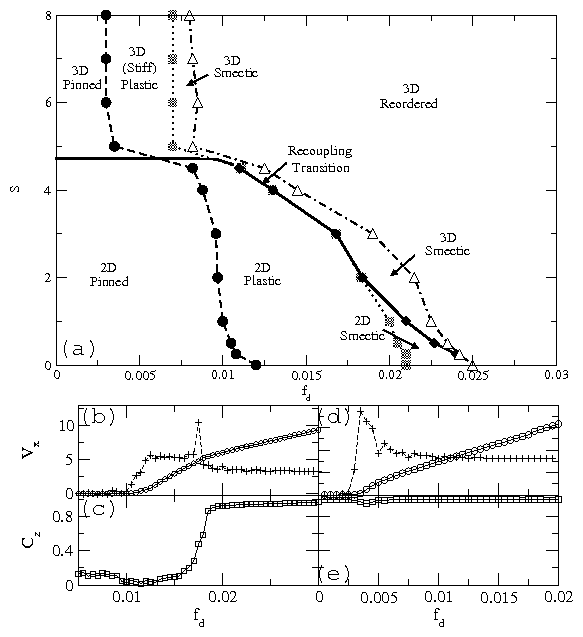
 Figure 1: (a) Phase diagram for varying interlayer coupling s and driving
force fd. Circles: depinning line; diamonds: decoupling line;
squares: smectic transition line; triangles: recrystallization line.
(b) Vx (circles) and dV/dI (+ signs) for s=2.0.
(c) Cz for s=2.0
(d) Vx (circles) and dV/dI (+ signs) for s=8.0.
(e) Cz for s=8.0
Figure 1: (a) Phase diagram for varying interlayer coupling s and driving
force fd. Circles: depinning line; diamonds: decoupling line;
squares: smectic transition line; triangles: recrystallization line.
(b) Vx (circles) and dV/dI (+ signs) for s=2.0.
(c) Cz for s=2.0
(d) Vx (circles) and dV/dI (+ signs) for s=8.0.
(e) Cz for s=8.0
|
