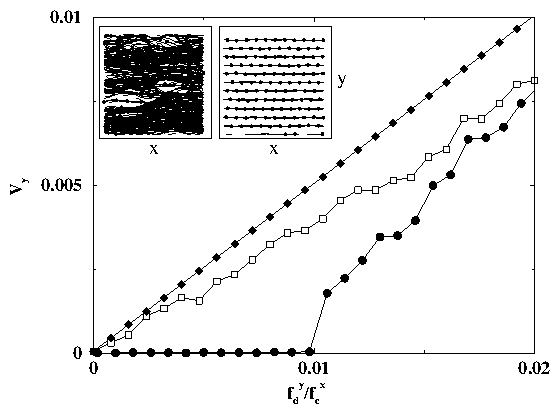
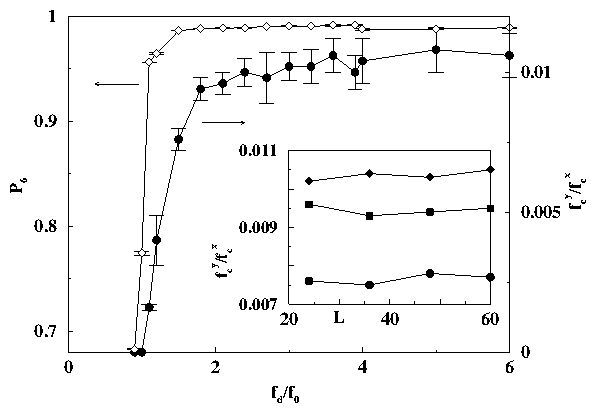
 Figure 2: Circles: Transverse critical force fcy/fcx versus
longitudinal driving force fd. Diamonds: Corresponding fraction of
six-fold coordinated vortices, P6. The transverse critical force
saturates for a reordered vortex lattice, fd/f0 > 1.8,
but drops as the
lattice becomes defected, reaching zero at fd/f0 ≈ 1.0.
Inset: Transverse critical force for different
system sizes L=24λ, 36λ, 48λ, and 60λ.
Circles: fd/f0 = 1.5; Squares: fd/f0 = 2.0; Diamonds:
fd/f0 = 3.0.
Figure 2: Circles: Transverse critical force fcy/fcx versus
longitudinal driving force fd. Diamonds: Corresponding fraction of
six-fold coordinated vortices, P6. The transverse critical force
saturates for a reordered vortex lattice, fd/f0 > 1.8,
but drops as the
lattice becomes defected, reaching zero at fd/f0 ≈ 1.0.
Inset: Transverse critical force for different
system sizes L=24λ, 36λ, 48λ, and 60λ.
Circles: fd/f0 = 1.5; Squares: fd/f0 = 2.0; Diamonds:
fd/f0 = 3.0.
|