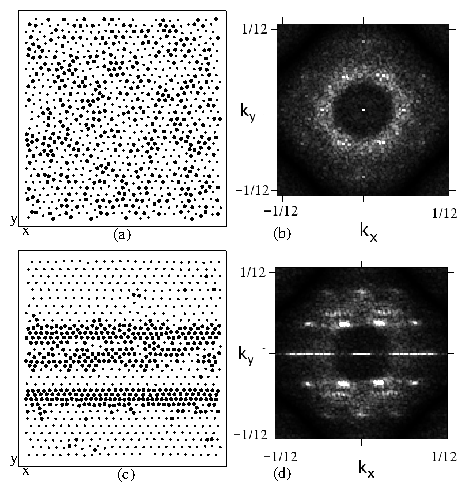
 Figure 1:
A bidisperse system of colloids with qA/qB=3 and n=0.385/a02.
(a,c) Real space colloid configurations. Small circles: species A;
large circles: species B.
(b,d) The corresponding structure factor S(k).
(a,b) Equilibrium state with no pinning
or driving. (c,d) Moving stripe state for Fp = 1.0, np=4.0/a02, and
FD/FC = 3.0.
Figure 1:
A bidisperse system of colloids with qA/qB=3 and n=0.385/a02.
(a,c) Real space colloid configurations. Small circles: species A;
large circles: species B.
(b,d) The corresponding structure factor S(k).
(a,b) Equilibrium state with no pinning
or driving. (c,d) Moving stripe state for Fp = 1.0, np=4.0/a02, and
FD/FC = 3.0.
|
