
  
|
|
2005 LDRD DR PRE-PROPOSAL (pdf)
Lagrangian Fluid Measurements and Turbulent Mixing
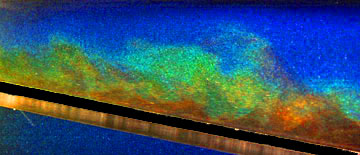
Experimental image of interfacial mixing of gravity current
down an incline -
color is proportional to density
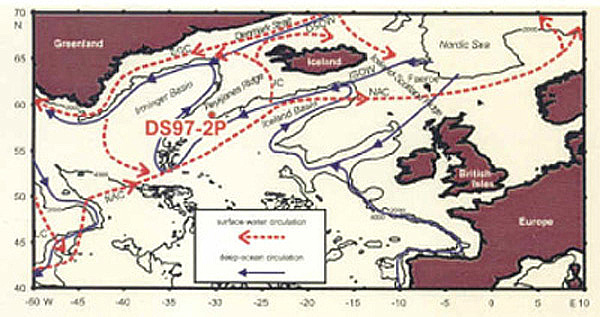
Map of the currents in the North Atlantic
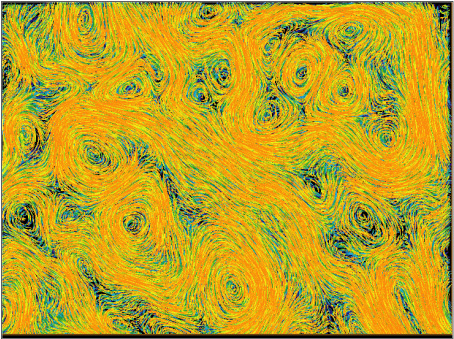
Color-coded image of particle tracks in a two-dimensional
stratified layer system.

Particle trajectories of individual particles tracked
as in image above.
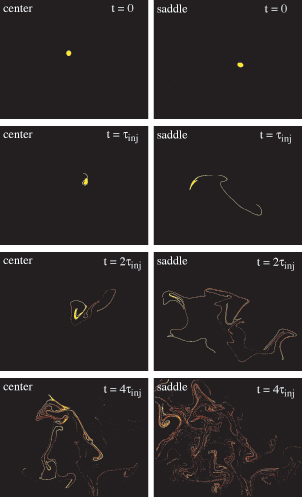
Evolution (in units of Lagrangian correlation time) of
a passive scalar advected by the experimentally determined velocity field.
Initial deposition of passive scalar was inside a center (elliptic) region
for sequence on left and inside a saddle (hyperbolic) region for the
sequence on right.
| Team Staff Members |
Robert
Ecke (PI, MST-10,cv): Fluid dynamics, particle image/tracking
velocimetry, thermochromic temperature field visualization, filter-approach
data analysis of energy/enstrophy transfer, flow topology. |
| Beth Wingate (Co-PI, CCS-2): |
Brad Holian (T-12) |
| Misha Chertkov (T-13): |
Daniel Livescu (CCS-2) |
| Matthew Hecht (CCS-2): |
Mike Brown (D-4) |
| Timothy Germann (X-7) |
Darryl Holm (T-7): |
| David Sharp (T-13) |
Rainer Bleck (EES-2): |
| Postdoctoral Fellows |
External Collaborators |
- Brent Daniel
(MST-10)
- Michael Rivera (MST-10)
- Susan Kurien (T-7)
|
|
Publications
| Ocean dynamics modeling |
- An isopycnic model study of the North-Atlantic. 1.
Model experiment, A. New, R. Bleck, Y. Jia, R. Marsh,
M. Huddleston, and S. Barnard, J. Phys. Ocn. 25,
2667 (1995).
- An isopycnic model study of the North Atlantic. 2. Inderdecadal
variability of the subtropical gyre, A. New and R. Bleck,
J. Phys. Ocngr. 25, 2700 (1995).
- The influence of layer outcropping of the separation
of boundary currents. 2. The wind-driven and buoyancy-driven experiments,
E. Chassignet, R. Bleck, and C. Rooth, J. Phys. Ocngr.
25, 2404 (1995).
- A comparison of data-parallel and message-passing versions
of the Miami isopycnic coordinate ocean model (MICOM),
R. Bleck, S. Dean, M. Okeefe M and A. Sawdey, Parall. Comp.
21, 1695 (1995).
- Upwind-wieghted advection schemes for ocean tracer transport:
An evaluation in a passive tracer context, M. Hecht,
W Holland, and P. Rasch, J. Geophys. Res.-Ocn. 100,
20763 (1995).
- A model comparison: Numerical simulations of the North
and equatorial Atlantic Oceanic circulation in depth and isopycnic
coordinates, E. Chassignet, L. Smith, R. Bleck, and F.
Bryan, J. Phys. Ocn. 26, 1849 (1996).
- Multidimensional forward-in-time and upstream-in-space-based
differencing for fluids, H. Drange and R. Bleck, Mon.
Wthr. Rev. 125, 616 (1997).
- Inclusion of thermobaricity in isopycnic-coordinate
ocean models, S. Sun, R. Bleck, C. Rooth, J. Dukowicz,
E. Chassignet, and P. Killworth, J. Phys. Ocngr. 29,
2719 (1999).
- A new approximation of the equation of state for seawater,
suitable for numerical ocean models,
D. Brydon, S. Sun and R. Bleck, J. Geophys. Res.-Ocns.
104 1537 (1999).
- Turbulent behavior of a fine mesh (1/12 degrees) numerical
simulation of the North Atlantic, A. Paiva, J. Hargrove,
E. Chassignet, and R. Bleck, J. Mar. Sys. 21,
307 (1999).
- The impact of lateral boundary conditions and horizontal
resolution on North Atlantic water mass transformations and pathways
in an isopycnic coordinate ocean model, L. Smith, E.
Chassignet, and R. Bleck, J. Phys. Ocngr. 30,
137 (2000).
- Sea surface velocities from sea surface temperature
image sequences 1. Method and validation using primitive equation
model output, X. Vigan, C. Provost, R. Bleck R, and P.
Courtier, J. Geophys. Res.-Ocn. 105
(2000).
- Numerical simulation of the North Atlantic Ocean at
1/10 degrees, R. Smith, M. Maltrud, R. Bryan and M. Hecht,
J. Phys. Ocngr. 30, 1532 (2000).
- Atlantic thermohaline circulation and its response to
increasing CO2 in a coupled atmosphere-ocean model, S.
Sun S and R. Bleck, Geophys. Res. Lett. 28,
4223 (2001).
- Thermohaline circulation studies with an Isopycnic
Coordinate Ocean Model
S. Sun S and R. Bleck, J. Phys. Ocngr. 31,
2761 (2001).
|
| Two-Dimensional & Quasi-geostrophic Flows |
- Turbulence in flowing soap films: Velocity, vorticity, and
thickness fields, M. Rivera, P. Vorobieff, R.E. Ecke, Phys.
Rev. Lett. 81, 1417 (1998).
- External dissipation in driven two-dimensional turbulence,
M. Rivera and X. Wu, Phys. Rev. Lett. 85,
976 (2000).
- Homogeneity and the inertial range in driven two-dimensional
turbulence, M. Rivera and XL. Wu,
Phys. Fluids 14, 3098 (2002).
- Soap film flows: Statistics of two-dimensional turbulence,
P. Vorobieff, M. Rivera and R. Ecke, Phys. Fluids 11,
2167 (1999).
|
| Mathematical Analysis |
- Tensor product Gauss-Lobatto points are Fekete points
for the cube, L. Bos, M. Taylor and B. Wingate, Math.
Comp. 70, 1543 (2001).
- A generalized diagonal mass matrix spectral element
method for non-quadrilateral elements,
M. Taylor and B. Wingate, Appl. Num. Math. 33,
259 (2000).
- Wave propagation in 2-D elastic media using a spectral
element method with triangles and quadrangles, D. Komatitsch,
R. Martin, J. Tromp, M. Taylor and B. Wingate, J. Comp. Acous.
9, 703 (2001).
- An algorithm for computing Fekete points in the triangle,
M. Taylor, B. Wingate, and R. Vincent,
SIAM J. Numer. Anal. 38, 1707 (2000).
- On simulating flows with multiple time scales using
a method of averages, B. Nadiga, M. Hecht, L. Margolin
and P. Smolarkiewicz, Theor. Comp. Fluid Dyn. 9,
281 (1997).
|
| Experimental Fluid Dynamics |
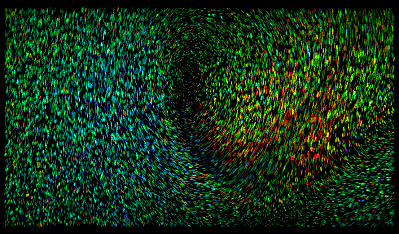
Buoyant
downwelling convective plume - Colors indicate
local
temperature and colored line segments represent local fluid velocity.

Expanded
region around the thermal plume
- Excitation of spirals and chiral-symmetry breaking in Rayleigh-Benard
convection, R. Ecke, Y. Hu, R. Mainieri, and G. Ahlers, Science
269, 1704 (1995).
- Time and Length Scales in Rotating Rayleigh-Benard Convection,
Y.C. Hu, R.E. Ecke, and G. Ahlers, Phys. Rev. Lett.
74, 5040 (1995).
- Heat transport scaling in turbulent Rayleigh-Benard convection:
Effects of rotation and Prandtl number, Y. Liu and R. Ecke,
Phys. Rev. Lett. 79, 2257 (1997).
- Mechanisms of extensive spatiotemporal chaos in Rayleigh-Bernard
convection, D. Egolf, I. Melnikov, W. Pesch, and R. Ecke,
Nature 404, 733 (2000).
- Does turbulent convection feel the shape of the container?
Z. Daya and R. Ecke, Phys. Rev. Lett. 8718,
4501 (2001).
- Turbulent rotating convection: An experimental study,
P. Vorobieff and R. Ecke, J. Fluid Mech. 458, 191 (2002).
Energy and enstrophy transfer in two-dimensional turbulence,
M. Rivera, W. Daniel and R. Ecke, Phys. Rev. Lett. (2003).
|
| Subgrid- Modeling Theory |
- Long-time shallow-water equations with a varying bottom,
R. Camassa, D. Holm, and C. Levermore, J. Fluid Mech.
349, 173 (1997).
- Camassa-Holm equations as a closure model for turbulent
channel and pipe flow,
S. Chen, C. Foias, D. Holm, E. Olson, E. Titi, and S. Wynne, Phys.
Rev. Lett. 81, 5338 (1998).
|
| Theory |
|
|
| Particle Tracking/Image Velocimetry |
| |
|
