Granular Media

Granular media is unique as it involve macroscopically sized particles and large energy dissipation. Yet, they can exhibit solid-like or liquid-like behavior. They also exhibit interesting collective phenomena such size segregation, pattern formation, shock waves, slow density relaxation, and density inhomogeneities.

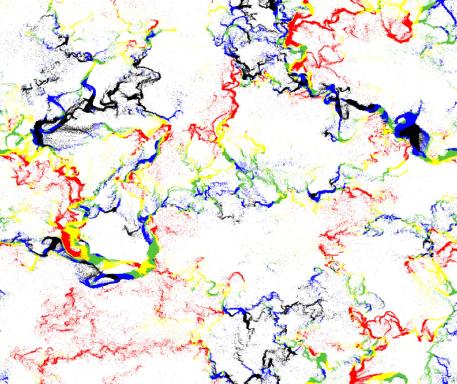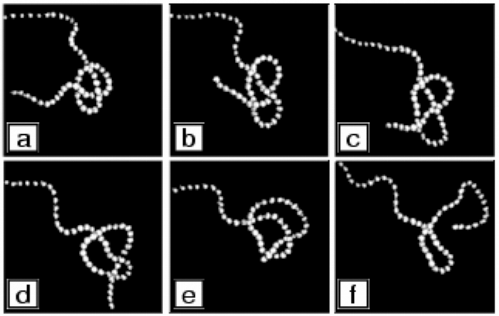
Knots in Granular Chains: (see also quiktime movie (5MB), wmv movie (20MB!), mpeg movie (32MB!), experimental data, pictures, poster): Statisticalproperties of the opening times of knots in vertically vibrated granular chains were studied experimentally and theoretically. The measurements are in good qualitative and quantitative agreement with a theoretical model involving three random walks interacting via hard core exclusion in one spatial dimension. In particular, the knot survival probability follows a universal scaling function which is independent of the chain length, with a corresponding diffusive characteristic time scale. Both the large-exit-time and the small-exit-time tails of the distribution are suppressed exponentially, and the corresponding decay coefficients are in excellent agreement with the theoretical values. The figure shows illustrative snapshots of the vibrated knot experiment, taken at equally spaced time intervals corresponding to ten plate oscillation cycles.

Density Inhomogeneities: (see also poster) Granular flow is typically modeled using dissipative (inelastic) gases. We have shown that the dynamics of such gases in one-dimension is universal, i.e., it is independent of the degree of inelasticity. Furthermore, the clustering and all other asymptotic properties such as the velocity distribution are described by a classical theory, the Burgers equation, which is also used to model "sticky gases" in the context of large scale formation of matter in the universe. Below is a comparison in two-dimensions between the the vector field (darker areas indicate larger gradients and hence, densities) in the Burgers equation and MD simulations.

Kinetic Theory: (see also poster) An analytical solution for the moments of the velocity distribution in a kinetic theory of inelastic collision processes with constant (Maxwell) collision kernel shows a multiscaling asymptotic behavior. This implies an infinite hierarchy of relaxation time scales underlying the distribution. In turn, this hierarchy of time scales is directly related to the cummulants of the steady state distribution of the same system in the presence of a heat bath, a sort of generalized fluctuation dissipation relations. Additionally, compact velocity distribution develop an infinite set of progressively weaker singularities.
Phase Transitions: Experimental studies of vibrated granular monolayers by J.S.Urbach and J.S.Olafsen (Georgetown Univ.) show vibrated granular monolayers undergo an order-disorder transition. We used molecular dynamics simulations to study details of this transition. At high excitation strengths, the system is in a gas state, particle motion is isotropic, and the velocity distributions are Gaussian. As the vibration strength is lowered the system's dimensionality is reduced from three to two. Below a critical excitation strength, a gas-cluster phase occurs, and the velocity distribution becomes bimodal. In this phase, the system consists of clusters of immobile particles arranged in close-packed hexagonal arrays, and gas particles whose energy equals the first excited state of an isolated particle on a vibrated plate. The figures compare simulation (color figs) versus experiment (b/w figs).

Compaction: (see also poster): Experimental studies show that the density of a vibrated granular material evolves from a low density initial state into a higher density final steady state. The relaxation towards the final density follows an inverse logarithmic law. As the system approaches its final state, a growing number of grains have to be rearranged to enable a local density increase. The time for such rearrangement diverges exponentially thereby leading to extremely slow relaxation. An analytically tractable one-dimensional parking model model mimics this behavior and is also useful for understanding experimentally observed steady state density fluctuations. 
Flow: Describing the flow properties of granular materials is especially difficult due to their strong dissipative nature. This leads to large density inhomogeneities and steady states that are nonequilibrium in nature. For example, if we introduce an inhomogeneous energy input, a granular system achieves a steady state in which there are "hot" dilute and "cold" dense regions. This behavior was observed recently in experimental as well as theoretical/numerical studies of inelastic gases. In the figure, the left wall was kept at fized temperature and all other three walls are elastic.